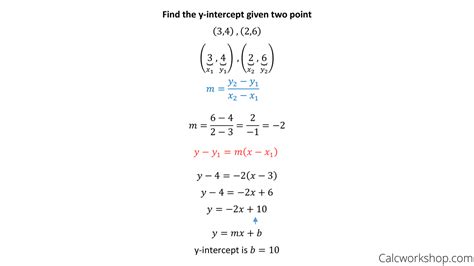
To find the y-intercept of a line given two points, we can use the slope-intercept form of a linear equation, which is y = mx + b, where m is the slope of the line, and b is the y-intercept. The y-intercept is the point at which the line crosses the y-axis, and it's a crucial piece of information for understanding the behavior of the line.
Naturally Finding the Y Intercept

The first step in finding the y-intercept with two points is to calculate the slope (m) of the line. The formula for slope given two points (x1, y1) and (x2, y2) is m = (y2 - y1) / (x2 - x1). This formula calculates the rise over run, which gives us the steepness of the line. For example, if our two points are (1, 2) and (3, 4), the slope would be m = (4 - 2) / (3 - 1) = 2 / 2 = 1. This means for every unit the line moves to the right, it moves up one unit.
Applying the Slope-Intercept Form
Once we have the slope, we can substitute one of the points into the slope-intercept form of the equation to solve for b, the y-intercept. Using the point (1, 2) and the slope m = 1, we plug these values into y = mx + b, which gives us 2 = 1(1) + b. Simplifying this equation, we get 2 = 1 + b, and solving for b, we subtract 1 from both sides, which yields b = 1. Therefore, the y-intercept of the line is 1, meaning the line crosses the y-axis at the point (0, 1).
| Point | X Coordinate | Y Coordinate |
|---|---|---|
| 1 | 1 | 2 |
| 2 | 3 | 4 |
| Y-Intercept | 0 | 1 |

Key Points
- The slope-intercept form of a line (y = mx + b) is used to find the y-intercept, where m is the slope, and b is the y-intercept.
- The slope (m) of a line given two points (x1, y1) and (x2, y2) is calculated using the formula m = (y2 - y1) / (x2 - x1).
- Once the slope is known, substituting one of the given points into the equation y = mx + b allows solving for b, the y-intercept.
- The y-intercept is the point where the line crosses the y-axis and is represented as (0, b).
- Understanding the y-intercept is vital for graphing lines and analyzing linear relationships in various contexts, including physics, economics, and more.
In conclusion, finding the y-intercept with two points involves calculating the slope of the line using the two given points and then applying the slope-intercept form of the linear equation. This method provides a straightforward approach to determining the y-intercept, which is a fundamental aspect of linear equations and their graphical representations.
What is the significance of the y-intercept in a linear equation?
+The y-intercept is significant because it tells us where the line crosses the y-axis, providing crucial information about the line’s position and its intercept with the y-axis.
Can the y-intercept be negative?
+Yes, the y-intercept can be negative. A negative y-intercept means the line crosses the y-axis below the origin (0,0), indicating that the line has a negative value when x equals 0.
How does the y-intercept relate to real-world applications?
+The y-intercept has numerous real-world applications, including physics, where it can represent initial conditions, and economics, where it can represent a baseline or starting point for models and forecasts.