The Maclaurin series, a fundamental concept in calculus, is a powerful tool for approximating functions and understanding their behavior. Named after the Scottish mathematician Colin Maclaurin, who introduced the concept in the 18th century, the Maclaurin series is a specific type of Taylor series that is centered at zero. This series is crucial for a variety of applications, including physics, engineering, and computer science, where it is used to model and analyze complex phenomena. Understanding the Maclaurin series requires a solid foundation in calculus and an appreciation for the intricacies of mathematical modeling.
Key Points
- The Maclaurin series is a Taylor series centered at zero, providing a way to approximate functions around the origin.
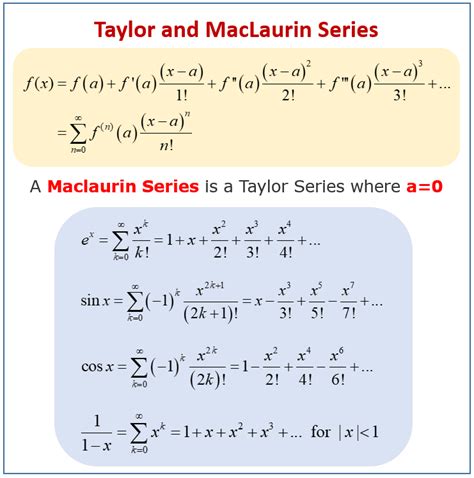
- It is defined by the formula $f(x) = f(0) + f'(0)x + \frac{f''(0)}{2!}x^2 + \frac{f'''(0)}{3!}x^3 + \cdots$.
- Calculating the Maclaurin series involves finding the derivatives of the function at zero and applying them in the series formula.
- Convergence of the series is crucial; it must be determined whether the series converges to the original function over a specific interval.
- Practical applications of the Maclaurin series include approximating transcendental functions, solving differential equations, and modeling physical systems.
Understanding the Maclaurin Series Formula

The Maclaurin series formula is given by f(x) = f(0) + f'(0)x + \frac{f''(0)}{2!}x^2 + \frac{f'''(0)}{3!}x^3 + \cdots + \frac{f^{(n)}(0)}{n!}x^n + \cdots. This formula represents the function f(x) as an infinite sum of terms, where each term involves a derivative of f evaluated at x=0, multiplied by x raised to a power that corresponds to the order of the derivative, and divided by the factorial of that power. Understanding this formula is key to applying the Maclaurin series in various mathematical and scientific contexts.
Calculating the Maclaurin Series for Common Functions
One of the most common applications of the Maclaurin series is in approximating transcendental functions such as e^x, \sin(x), and \cos(x). For example, the Maclaurin series for e^x is 1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \cdots, which is derived by calculating the derivatives of e^x at x=0 and applying them in the Maclaurin series formula. Similarly, the series for \sin(x) is x - \frac{x^3}{3!} + \frac{x^5}{5!} - \cdots, obtained by evaluating the derivatives of \sin(x) at zero and substituting into the formula.
| Function | Maclaurin Series |
|---|---|
| $e^x$ | $1 + x + \frac{x^2}{2!} + \frac{x^3}{3!} + \cdots$ |
| $\sin(x)$ | $x - \frac{x^3}{3!} + \frac{x^5}{5!} - \cdots$ |
| $\cos(x)$ | $1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \cdots$ |
Practical Applications and Limitations

The Maclaurin series has numerous practical applications, ranging from approximating functions that are difficult to compute directly, to solving differential equations, and modeling physical systems. However, it is also important to consider the limitations of the Maclaurin series, including the potential for slow convergence or divergence for certain functions or values of x. Understanding these limitations is crucial for applying the Maclaurin series effectively in real-world problems.
Addressing Common Challenges
One of the common challenges in working with the Maclaurin series is determining the interval of convergence. This can be addressed by applying convergence tests, such as the ratio test or the root test, to the series. Another challenge is the computational effort required to calculate higher-order derivatives, which can be mitigated by using computational tools or identifying patterns in the derivatives of common functions.
What is the primary use of the Maclaurin series in mathematics and physics?
+The primary use of the Maclaurin series is to approximate functions and understand their behavior around the origin. It is particularly useful for approximating transcendental functions and solving differential equations.
How do you determine the interval of convergence for a Maclaurin series?
+The interval of convergence can be determined using the ratio test or the root test. These tests involve calculating the limit of the ratio or root of successive terms in the series as $n$ approaches infinity.
What are some common applications of the Maclaurin series in engineering and computer science?
+The Maclaurin series is used in engineering and computer science for a variety of applications, including modeling physical systems, solving differential equations, and approximating complex functions. It is also used in numerical analysis and computational methods.
In conclusion, the Maclaurin series is a fundamental tool in mathematics and science, offering a powerful method for approximating functions and understanding their behavior. By mastering the Maclaurin series and its applications, individuals can gain a deeper insight into the workings of complex systems and phenomena, and develop practical skills for solving problems in a variety of fields. Whether in physics, engineering, computer science, or pure mathematics, the Maclaurin series remains an indispensable resource for anyone seeking to model, analyze, and understand the world around us.



