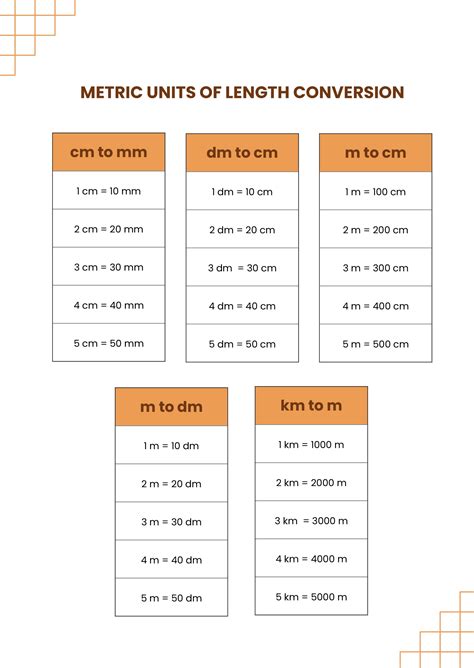
Unit conversion is a fundamental aspect of various scientific, engineering, and everyday applications, enabling the transformation of quantities from one unit of measurement to another. This process is crucial for ensuring accuracy, consistency, and reliability in calculations and data analysis. However, it can often be challenging, especially when dealing with complex units or systems. To navigate these challenges effectively, it's essential to understand the basics of unit conversion and to employ strategies that simplify the process. Here, we'll explore five key tips for mastering unit conversion, making it easier to work with different units of measurement across various disciplines.
Key Points
- Understanding the unit conversion process involves recognizing the relationship between different units of measurement.
- Familiarity with conversion factors is crucial for accurate conversions.
- Using dimensional analysis can simplify complex conversions by canceling out units.
- Practicing with real-world examples helps in developing proficiency in unit conversion.
- Double-checking calculations for unit consistency and numerical accuracy is vital.
Tip 1: Mastering Conversion Factors

Conversion factors are ratios of equivalent quantities, expressed in different units. For instance, knowing that 1 inch equals 2.54 centimeters is a conversion factor. These factors are essential for converting between units within the same system (like inches to feet) or between different systems (such as metric to imperial). To master conversion factors, it’s helpful to memorize common ones or keep a reference handy. When applying conversion factors, ensure that the units cancel out appropriately, leaving you with the desired unit for your result.
Application of Conversion Factors
Applying conversion factors involves setting up ratios that allow units to cancel out, leaving the desired unit. For example, to convert 10 meters into feet, knowing that 1 meter equals approximately 3.281 feet, you set up the conversion as follows: 10 meters * (3.281 feet / 1 meter) = 32.81 feet. This step ensures that the meter units cancel out, leaving the result in feet.
Tip 2: Understanding Dimensional Analysis

Dimensional analysis is a method used in physics and engineering to simplify complex physical expressions by canceling out units. This technique is based on the principle that physical quantities can be expressed as a product of a numerical value and a unit. By applying dimensional analysis, you can ensure that your calculations are unit-consistent, which is critical for obtaining meaningful and accurate results. The process involves setting up equations so that units in the numerator and denominator cancel out appropriately, leaving the desired unit for the result.
Example of Dimensional Analysis
An example of dimensional analysis in action is converting the speed of a car from kilometers per hour to meters per second. If a car travels at 100 kilometers per hour, and knowing that 1 kilometer equals 1000 meters and 1 hour equals 3600 seconds, you can convert the speed as follows: 100 kilometers/hour * (1000 meters / 1 kilometer) * (1 hour / 3600 seconds) = 100 * 1000 / 3600 meters/second = 27.78 meters/second. This calculation demonstrates how dimensional analysis simplifies unit conversions by canceling out units systematically.
Tip 3: Practicing with Real-World Examples
Practicing unit conversions with real-world examples is one of the most effective ways to become proficient. Real-world scenarios often present complex conversion challenges that require a deep understanding of units and their relationships. For instance, calculating the area of a room in square meters when the dimensions are given in feet, or determining the volume of water in liters from a measurement in gallons. By working through such examples, you develop the ability to apply unit conversion principles to practical problems, enhancing your competence in handling different measurement systems.
Benefits of Practical Application
The practical application of unit conversion principles offers several benefits. It helps in reinforcing theoretical knowledge, improving problem-solving skills, and developing a deeper understanding of how different units relate to each other. Moreover, real-world examples often involve mixed units or require conversions between different systems, which helps in building flexibility and adaptability in handling various measurement challenges.
Tip 4: Checking for Unit Consistency
Ensuring that units are consistent throughout calculations is critical for accuracy. A mistake in unit conversion can lead to incorrect results, which might have significant implications in scientific research, engineering designs, or everyday applications. Therefore, it’s essential to double-check that the units of the result match the desired units and that the conversion process has been applied correctly. This verification step involves reviewing the calculation to ensure that all units have been properly canceled out or converted, leaving the result in the appropriate units.
Importance of Verification
Verifying unit consistency is not just about accuracy; it’s also about ensuring the reliability and reproducibility of results. In scientific and engineering contexts, results must be precise and trustworthy, as they often form the basis for further research, design decisions, or policy implementations. By meticulously checking unit conversions, professionals can guarantee that their work meets the highest standards of quality and reliability.
Tip 5: Embracing Technology and Resources
In today’s digital age, there are numerous tools and resources available to assist with unit conversions, ranging from online conversion calculators to mobile apps and software programs. These tools can significantly simplify the conversion process, especially for complex or less common units. Moreover, many scientific calculators and computer algebra systems have built-in functions for unit conversions, making it easier to perform conversions accurately and efficiently. Embracing these technologies and resources can enhance productivity and reduce the likelihood of errors in unit conversion tasks.
| Conversion Type | Conversion Factor |
|---|---|
| Length: meters to feet | 1 meter = 3.281 feet |
| Volume: liters to gallons | 1 liter = 0.2642 gallons |
| Mass: kilograms to pounds | 1 kilogram = 2.2046 pounds |

What is the most common mistake in unit conversion?
+One of the most common mistakes in unit conversion is failing to ensure that the units cancel out properly, leading to incorrect results. This can be avoided by carefully setting up conversion factors and verifying that the units of the result match the desired units.
How can I improve my skills in unit conversion?
+Improving your skills in unit conversion involves practice, familiarity with common conversion factors, and understanding the principles of dimensional analysis. Working through real-world examples and using technology and resources available can also enhance your proficiency.
What are some common units that are often confused in conversions?
+Units that are often confused in conversions include meters and feet for length, liters and gallons for volume, and kilograms and pounds for mass. Ensuring accuracy in these conversions is crucial, especially in applications where precision is key.