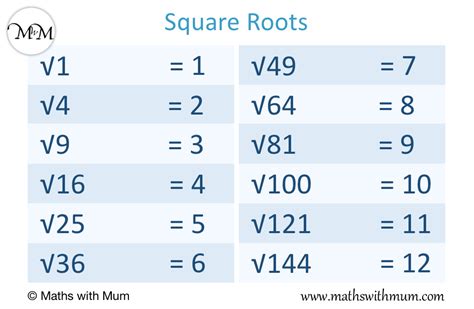
The square root of 1 is a fundamental concept in mathematics, and its simplicity can often lead to oversights in understanding its significance. At its core, the square root of a number is a value that, when multiplied by itself, gives the original number. In the case of the square root of 1, this means that the result of the multiplication of this value by itself equals 1. This is represented mathematically as √1 = x, where x is the number that, when squared (x^2), equals 1.
Mathematically, there are two solutions to the equation x^2 = 1: x = 1 and x = -1. This is because both 1 squared (1^2) and -1 squared (-1^2) equal 1. The positive square root of 1 is 1, and the negative square root of 1 is -1. This concept is crucial in various mathematical operations and applications, including algebra, geometry, and calculus, where understanding the nature of square roots and their implications is essential for solving equations and analyzing functions.
Key Points
- The square root of 1 has two solutions: 1 and -1, because both 1^2 and (-1)^2 equal 1.
- Understanding the square root of 1 is fundamental in mathematics, affecting concepts in algebra, geometry, and calculus.
- The positive square root of 1 is defined as 1, and the negative square root of 1 is defined as -1.
- These definitions are based on the principle that the square of a number (whether positive or negative) results in a positive value.
- The concept of square roots, including that of 1, underpins more complex mathematical theories and applications, such as solving quadratic equations and analyzing quadratic functions.
Mathematical Implications and Applications

The square root of 1, with its dual solutions, has profound implications in mathematics. It serves as a foundational element in algebra, particularly in solving quadratic equations. A quadratic equation is of the form ax^2 + bx + c = 0, where a, b, and c are constants, and x is the variable. The solutions to such equations can involve square roots, and understanding that the square root of 1 has two values informs how we interpret and solve these equations.
In geometry, the concept of square roots, including the square root of 1, plays a role in calculating distances and understanding spatial relationships. The Pythagorean theorem, for example, which states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides, relies on the concept of square roots for its application.
Algebraic and Geometric Interpretations
Algebraically, the square root of 1 is crucial in simplifying expressions and solving equations. For instance, in the equation x^2 - 1 = 0, factoring it into (x - 1)(x + 1) = 0 yields solutions x = 1 and x = -1, illustrating the dual nature of the square root of 1. Geometrically, this concept translates into understanding reflections and symmetries in graphs and geometric shapes. The fact that -1 is also a square root of 1 highlights the symmetry inherent in many mathematical structures.
| Mathematical Operation | Example | Result |
|---|---|---|
| Squaring 1 | 1^2 | 1 |
| Squaring -1 | (-1)^2 | 1 |
| Factoring x^2 - 1 | (x - 1)(x + 1) = 0 | x = 1 or x = -1 |

Advanced Mathematical Concepts and the Square Root of 1

As one delves deeper into mathematics, the concept of the square root of 1 becomes integral to understanding more advanced theories. In calculus, for example, the derivative of a function represents the rate of change of the function’s output with respect to one of its variables. The concept of limits, which underlies calculus, relies on the understanding of how functions behave as their input values approach certain points, including understanding the behavior of square root functions.
In complex analysis, a branch of mathematics that deals with functions of complex numbers, the square root of 1 plays a role in defining complex square roots and understanding the properties of complex functions. The complex numbers are an extension of the real numbers, including an imaginary unit i, where i^2 = -1. This extension allows for the square root of negative numbers to be defined, further expanding the realm of mathematical operations and applications.
Complex Numbers and Square Roots
The introduction of complex numbers expands the concept of square roots, allowing for the square roots of negative numbers to be defined. For instance, the square root of -1 is represented by the imaginary unit i, where i^2 = -1. This concept is crucial in many fields, including engineering, physics, and computer science, where complex numbers are used to model and analyze systems that exhibit periodic behavior, such as electrical circuits and mechanical vibrations.
In conclusion, the square root of 1, though seemingly straightforward, holds significant importance in the foundation and application of mathematical concepts. Its dual nature, being both 1 and -1, reflects the symmetry and complexity inherent in mathematical structures, influencing various branches of mathematics from algebra and geometry to calculus and complex analysis.
What is the square root of 1?
+The square root of 1 is 1 and -1 because both 1^2 and (-1)^2 equal 1.
Why is the concept of the square root of 1 important in mathematics?
+Understanding the square root of 1 is crucial for solving equations, analyzing functions, and applying mathematical concepts in various fields, including algebra, geometry, calculus, and complex analysis.
How does the concept of the square root of 1 relate to complex numbers?
+The concept of the square root of 1 extends into complex numbers, allowing for the definition of square roots of negative numbers, such as the imaginary unit i, where i^2 = -1.